Dee Newcum
dee.newcum@gmail.com
http://paperlined.org/dev/oss/high_energy_slings/
November 2011

This paper doesn't put much emphasis on accuracy. High-energy slings are often less accurate.[1]
In most situations, sling accuracy is more important than power. Ancient slings were probably used more often against single targets (hunting an animal, or an encounter with a single adversary), rather than scattershot at massed troops.
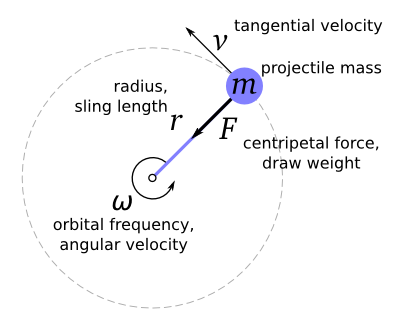 The kinetic energy of a slung projectile, expressed in terms of centripetal force on the sling, is [2]:
$$ E = {1 \over 2} r F $$
Where \(r\) is the radius (sling length), \(F\) is the centripetal force (analogous to a bow's draw weight), and \(E\) is the launch energy.
The kinetic energy of a slung projectile, expressed in terms of centripetal force on the sling, is [2]:
$$ E = {1 \over 2} r F $$
Where \(r\) is the radius (sling length), \(F\) is the centripetal force (analogous to a bow's draw weight), and \(E\) is the launch energy.
Expressing things in terms of draw weight is interesting, because it lets us ignore the details of projectile mass and orbital frequency.[3] At high draw weight, you start to have problems with the slinger not having enough arm strength to maintain a proper slinging technique, or the sling breaking. This is true regardless whether it's a light projectile that's rotating quickly, or a heavy projectile rotating slowly.
An example: One way to estimate the draw weight is to use weaker material of a known breaking strength, and confirm you can break it by using your normal slinging technique. We do this with a 10 foot sling made from 30lb test fishing line. The calculated projectile energy is ~200 joules when the sling breaks. (for Google Calculator, type in "1/2 * 10 feet * 30 pounds force")
This makes some intuitive sense. If the benefit of a sling is that it gives mechanical advantage — by lengthening a thrower's arm — then using a sling with more mechanical advantage might be useful in some circumstances.
However, aerodynamic drag is a significant limiting factor on sling length. The longer a sling is, the more the sling body slows the projectile down during spin-up, which ultimately limits maximum orbital frequency.
How do we solve this problem? The answer is to build the thinnest possible sling. Fishing line is easier to work with, but piano wire can be used as well.
The author learned this lesson the hard way. High forces focused on a thin line means that it can slice through muscle. After you get a lot of force on the line (>20lb draw weight), do not get your off-hand near it.
If you want to abort a launch, DO NOT USE YOUR OFF-HAND TO SLOW THE SLING DOWN. Instead, slow it down by using the opposite pumping movements — imagine that you're trying to get the projectile to spin in the opposite direction. If you're unsure how to do this, practice it before you get a sling spun up to maximum energy.
Another way to abort a launch is to dip the projectile near the ground so that it drags several times.
This warning especially applies to Spectra/Dyneema fishing line, because its soft pliability can fool you into thinking it's safer than piano wire. It's not. Spectra/Dyneema is factually stronger than steel.[4]
 Traditional slings use a pouch and a doubled-over line. In this paper, we explore non-traditional slings called "comet slings".[5] After release, the entire sling body remains attached to the projectile, and it follows the projectile through the air like a comet's tail.
Traditional slings use a pouch and a doubled-over line. In this paper, we explore non-traditional slings called "comet slings".[5] After release, the entire sling body remains attached to the projectile, and it follows the projectile through the air like a comet's tail.
This configuration has some benefits, compared to traditional slings:
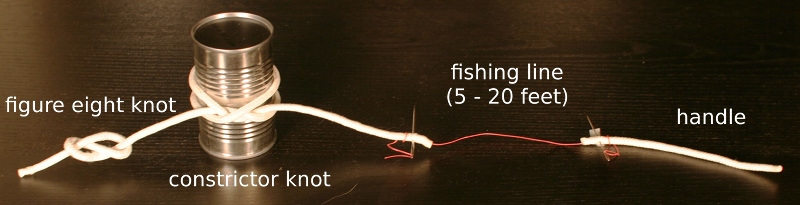

The strongest fishing line is made from braided Spectra/Dyneema.
Splice each end of the fishing line into the appropriate end-rope, using a needle. Stitch the fishing line through the rope several times. Take care to make sure this sewn connection is secure, since fishing line is slippery and it wants to unravel. Optionally, you can use any fishing knot as a backup knot.

By far, the biggest simplification is to completely replace the fishing line with thin paracord (thin and very strong rope). By using thin rope, you remove almost all problems associated with high-pressure line (where pressure means the same force concentrated on a much smaller area). There is no longer any need for separate end-ropes that need to be individually tied on. Safety is improved — there is no longer a risk of slicing through muscle. The obvious downside is that drag is increased, both during wind-up as well as mid-flight.
Another option is to keep the fishing line, but eliminate either or both of the end-ropes, and extend the fishing line all the way to the end.
Eliminating the projectile end-rope has a significant downside. The line takes a sharp 90° turn where the stem (the long straight portion that includes the fishing line) enters the constrictor knot. If you use fishing line at this point, this will be the weakest point of the system, and breaks will almost always happen here. Although Spectra/Dyneema is abrasion-resistant, it isn't as strong as rope at this junction, because rope spreads the force over a much larger area — the pressure on the rope is much less.
When using fishing line, using a detached handle has two benefits: 1) it simplifies construction, because one handle can be reused for many projectiles, and 2) drag is reduced during flight.
For lower pressures (either fishing line + low draw weight, or paracord), a leather glove is an acceptable "handle" (insofar as it protects your fingers from damage).
For higher pressures (fishing line + high draw weight), you can manufacture a handle. However, the rope really is adequate, particularly for larger projectiles where the effect of drag is comparatively less.
The author explored a number of different designs for a manufactured handle. The release/trigger mechanism proved to be tricky, and no good solution has been found yet. The author is still exploring.
Slingers use several different styles when using standard slings. However, helicopter style is the only viable technique for long slings, when standing on the ground.
If a pit, cliff, or tower can be located, and you can sling atop the tower or immediately adjacent to the pit, then other slinging styles may work. However, in a hunting or combat scenario, fixed towers/pits are usually unavailable. Even if they are available, they're tactically undesirable because they limit mobility.
A tip — during practice sessions, if you tie a 1" wide white ribbon near the handle end, this will make it easier to locate and retrieve projectiles. Without this, it is difficult to accurately see the impact position of the projectile from such a long distance away.
An example query for Google Calculator is .5 * (9.8 m / s^2) * 100 grams * 100 meters.
If the launch angle is known (e.g. by analyzing the frames of a video), then a more exact estimate can be obtained: [8] $$E = {m g d \over 2 sin(2 \theta)}$$

Hammer throw practitioners achieve a projectile energy of ~3000 joules, at the Olympic level.[10] [7] Working backwards, this translates to a draw weight of ~1100 lbs. [TODO: does this pass the smell test?]
We can calculate the energy of the entries listed on the slinging.org range records page:
| Slinger | Date | Sling type | Type | Mass | Throw style | Sling length | Range | Energy | Draw weight |
|---|---|---|---|---|---|---|---|---|---|
| Melvin Gaylor * | 1970 | 212.6g | 349.6m | ≥364.2J | |||||
| Vernon Morton * | 283.5g | 258.2m | ≥358.7J | ||||||
| SEB | Stone | 300g | Side-Arm | 130cm | ~220m | ≥323.4J | ≥111.9lbs | ||
| Colonel Walker | Orange | ~454g | Modified Underhand | 122cm | ~130m | ≥289.2J | ≥106.6lbs | ||
| Douglas | 02/11/05 | Heavy stone | ~500g | ~90m | ≥220.5J | ||||
| LoboHunter | 02/06/05 | Lead egg sinkers | 170g | Underhand | 109cm | 198.2m | ≥165.1J | ≥68.1lbs | |
| LoboHunter | 02/06/05 | Weighted golf balls | 162.5g | Underhand | 109cm | 196m | ≥156.1J | ≥64.4lbs | |
| Thomas | 02/15/05 | Softball | 312g | 95m | ≥145.2J | ||||
| David Engvall * | 1992 | Pouch-less, special release | Dart | 62g | 127cm | 477.0m | ≥144.9J | ≥51.3lbs | |
| David T | Cement ball | 164g | ~150m | ≥120.5J | |||||
| Larry Bray * | 1982 | Stone | 52g | 130cm | 437.1m | ≥111.4J | ≥38.5lbs | ||
| Naiyor | 09/05/11 | Lacrosse ball | ~142g | Underhand | 66cm | ~160m | ≥111.3J | ≥75.8lbs | |
| Saulius Pusinskas | 08/08/11 | Braided, leather pouch | Bipointed, cement | 100g | Pseudo Figure 8 | 90cm | 220m | ≥107.8J | ≥53.9lbs |
| Douglas | 02/11/05 | Lead gland | ~85g | ~250m | ≥104.1J | ||||
| FunSlinger | 06/05/11 | Stone | ~85g | Figure 8 | 208cm | ~219m | ≥91.2J | ≥19.7lbs | |
| Thomas | 02/15/05 | Hard baseball | 148g | 129cm | ~120m | ≥87.0J | ≥30.3lbs | ||
| SEB | 10/05/11 | Stone | ~100g | Underhand | 80cm | ~173m | ≥84.8J | ≥47.6lbs | |
| Sobieski | 02/11/11 | Stone | 90g | Side arm | 112cm | 180m | ≥79.4J | ≥31.9lbs | |
| Saulius Pusinskas | 08/08/11 | Braided, leather pouch | Stone | 70g | Pseudo Figure 8 | 90cm | 220m | ≥75.5J | ≥37.7lbs |
| LoboHunter | 02/06/05 | Egg-shaped stone | 85g | Underhand | 109cm | 177.3m | ≥73.8J | ≥30.5lbs | |
| Stephen Fitzgerald | 05/15/07 | 1" steel ball | 66g | 105cm | 212m | ≥68.6J | ≥29.4lbs | ||
| NonkinMonk | 03/01/05 | Stones | ~70g | 91cm | 182.9m | ≥62.7J | ≥31.0lbs | ||
| LoboHunter | 02/06/05 | Clay gland | 85g | Underhand | 109cm | 148.6m | ≥61.9J | ≥25.5lbs | |
| Stephen Fitzgerald | 03/13/06 | Smooth stone | ~70g | Oblique Greek | 105cm | ~180m | ≥61.7J | ≥26.4lbs | |
| Curious_Aardvark | 2007 | Stone | ~57g | Side-Arm | ~74cm | ~220m | ≥61.4J | ≥37.3lbs | |
| Crater_Caster | Stone | ~113g | Underhand | 60cm | ~107m | ≥59.2J | ≥44.4lbs | ||
| Colonel Walker | 10/15/05 | Stone | ~112g | Underhand | 64cm | ~107m | ≥58.7J | ≥41.3lbs | |
| Stephen Fitzgerald | 03/13/06 | Lead egg | 57g | Oblique Greek | 105cm | 200m | ≥55.9J | ≥23.9lbs | |
| Alsatian | 02/10/05 | ~90g | ~120cm | ~120m | ≥52.9J | ≥19.8lbs | |||
| Alsatian | 02/10/05 | ~90g | ~100cm | ~100m | ≥44.1J | ≥19.8lbs | |||
| Africa_Slinger | 06/05/11 | Golf ball | ~45g | Figure 8 | 107cm | ~200m | ≥44.1J | ≥18.5lbs | |
| Stephen Fitzgerald | 03/13/06 | Golf ball | 45g | Figure 8 | 105cm | ~190m | ≥41.9J | ≥17.9lbs | |
| Mike Greenfield | 04/05/05 | Stone | 82g | Nwmanitou's Overhand | 85cm | ~100m | ≥40.2J | ≥21.3lbs | |
| Leeds_Lobber | 05/20/05 | Lead ball | 42g | Side-Arm | 175cm | ~180m | ≥37.0J | ≥9.5lbs | |
| Jerzy Gasperowicz | 02/15/05 | Light stones | ~25g | ~250m | ≥30.6J | ||||
| Alsatian | 02/10/05 | ~90g | ~60cm | ~60m | ≥26.5J | ≥19.8lbs | |||
| Zorro | 09/05/11 | Spherical stone | ~40g | Overhead | 71cm | ~119m | ≥23.3J | ≥14.8lbs | |
| Naiyor | 07/05/11 | Salt flour glande | ~56g | Underhand | 66cm | ~80m | ≥22.0J | ≥15.0lbs | |
| LoboHunter | 02/06/05 | Foos ball | 42.5g | Underhand | 109cm | 88.2m | ≥18.4J | ≥7.6lbs | |
| MammotHunter | 02/15/05 | Bipointed, clay | 34g | 94cm | 101.5m | ≥16.9J | ≥8.1lbs | ||
| Peter van der Sluys | 10/16/07 | Fabric | lead, fishing weight | 15g | Helicopter | 76cm | 210m | ≥15.4J | ≥9.1lbs |
| Peter van der Sluys | 10/16/07 | Fabric | lead, fishing weight | 10g | Helicopter | 76cm | 200m | ≥9.8J | ≥5.8lbs |
| Peter van der Sluys | Woven hemp | Bipointed, clay | 11g | ~50cm | 119m | ≥6.4J | ≥5.8lbs | ||
| Peter van der Sluys | 10/16/07 | Fabric | Bipointed, clay | 6g | Helicopter | 76cm | 180m | ≥5.3J | ≥3.1lbs |
(details: \(r\)=10ft, projectile=1 lb grab hook, range=70m, \(\theta\)=~36° (based on the fact that I launched very close to the projectile scraping on the ground behind me))
However, if sling length is chosen to be as long as possible, just short of dragging on the ground, then sling length varies with launch angle (assuming helicopter style). With low launch angles, sling length can become much longer. So launch velocities increase with decreasing launch angle.
How does a varying launch velocity affect the optimum launch angle? The author's calculations indicate that lower launch angles are better for range, with very small launch angles being the best. See that page for caveats.
A computer physics simulator could be configured to help in exploring projectile shape and sling configuration.
Computer simulators are very useful for sling experiments. This is because there's so much variability in real-world field testing — different slingers have different skill levels, and most slingers use different projectiles and slings. Computer simulators eliminate this variability, and allow slingers from around the world to repeat the exact same experiment.
Simulators are also helpful, in that most slingers don't have the equipment to accurately measure how fast their projectiles are going, while measuring speeds and distances in a computer is cheap and easy.
All of the equations in this paper ignore air friction; a physics simulator would allow friction to be accounted for.
For an analysis of the kinematics of trebuchets, see Donald B. Siano's work at http://AlgoBeautyTreb.com/.
Also, when slingers increase orbital frequency, lateral accuracy gets decreased — release timing errors are exacerbated with increased orbital frequency.
That said, it is possible to retain accuracy while increasing energy. Range and orbital frequency can be kept the same, but sling length (and thus tangential velocity) and projectile mass can be increased, without impacting accuracy.
The centripetal force equation is: $$ F_{centrip} = {{m v^2} \over r}$$
Solve for velocity: $$ v = \sqrt { {r F_{centrip} } \over m }$$
Plug that into the kinetic energy equation, and we get: $$ E_{launch} = {1 \over 2} m v^2 = {1 \over 2} m \left({{r F_{centrip}} \over m}\right) = {1 \over 2} r F_{centrip}$$
An example Google Calculator query is "1 pound * 10 feet * (360 degrees / 1 second)^2 in pounds force".
Hammer throw practitioners spin their body while throwing, which significantly compromises their accuracy,[12] [TODO: source doesn't really support this conclusion] but allows them to use a hand position (wrists and elbows lax) that greatly increases their draw weight.
The author feels that the hammer throw technique compromises accuracy too much, so this paper focuses on a traditional slinging technique. Although we don't focus on accuracy in this paper, we don't want to prevent the possibility of being able to sling both accurately and powerfully.
The maximum projectile distance occurs with a launch angle of 45°, so we can omit the launch angle by introducing an inequality: $$d ≤ {v_0^2 \over g} sin(2 \cdot 45 ^{\circ} )$$ $$sin(90^{\circ} ) = 1$$ $$d ≤ {v_0^2 \over g}$$
Solve for \(v_0\): $$v_0 ≥ \sqrt {g d}$$
Plug that into the kinetic energy equation: $$E = {1 \over 2} m v_0^2$$ $$E ≥ {1 \over 2} m g d$$
These are licensed under a Creative Commons Attribution-ShareAlike 3.0 United States License.